How To Find Cartesian Equation
If polar equations have y'all second-guessing your future as a nuclear physicist, fret not! Near every pre-calculus student I have tutored has struggled here, and it isn't surprising at all. Remember the first fourth dimension you saw an equation and were introduced to these strange 10 and y variables? It may seem similar second nature at present, simply yous were learning about a whole new way to communicate about points and curves. Polar equations are no dissimilar. And I have good news! You already accept all the tools you need to learn to limited equations in polar course. In fact, you've been learning them for years; you have just been using them differently. Today, I'll discuss a foolproof method - Cambridge Coaching'due south V Step Process for converting polar to Cartesian equations. Polar coordinates exist to make information technology easier to communicate where a point is located. Permit'due south look at a quick example. Ignore the circles on the plot for a second and picture the rectangular system you're familiar with. Where would you lot put the betoken (three,4)? If you would put it by the red dot, yous're right. [image missing] Past at present, you know that the reddish dot tin can also be represented as (five,0.92) in polar coordinates. If nosotros wanted to motion the dot to the 30° line, while maintaining our distance of 5 units from the origin (the blue dot), we could simply limited it equally (5,thirty°) or (5,𝝅/half-dozen) in polar coordinates. If we were to express it in rectangular coordinates, the adding would require a few extra steps. And so, although polar coordinates seem to complicate things when you are first introduced to them, learning to use them tin can simplify math for yous quite a flake! Similarly, converting an equation from polar to rectangular form and vice versa can help you limited a curve more simply. Follow these five steps to convert equations between the polar and rectangular systems: A quick glance at your equation should tell you what form it is in.If it contains rs and θs, it is in polar form. If it contains xs and ys, it is in rectangular grade. If your equation is in polar class, your goal is to convert it in such a way that you lot are only left with xs and ys. If it is in rectangular form, your goal is to merely have rs and θs.It sounds uncomplicated, but reminding yourself of your goal volition assistance you avoid getting stuck half fashion through converting your equation (or going effectually in circles) . Now, accept a moment to examine your equation. Here are some key components you should exist looking for. If they are not present in your equation, you should be thinking about how you might be able to brand them appear. Bearing in mind the goal y'all set in Step ii, brainstorm to substitute. Simplify your equation by combining like terms. Where appropriate, mostly if you take x2s and y2s, think about completing the square. Where possible, a fully simplified equation will express r in terms of θ or y in terms of x, but this will sometimes exist impossible without truly ridiculous amounts of manipulation. Let's apply this method to a few examples. This is a polar equation. Our goal is to arrive at an equation that only contains x and y terms. Looking at the equation in a higher place, the right-manus side (RHS) could turn into rsin(θ), but is missing an r term. The left-hand side (LHS) could turn into 5r2, but is besides missing an r term. Aha! Since both the LHS and RHS are missing the same term, let's multiply both sides by r. 5r=sin(θ) 5r2=rsin(θ) Now that we accept an equation with terms that tin be converted easily, we tin can begin to substitute. 5r2=rsin(θ) 5(x2+y2)=y Finally, we combine like terms and simplify the equation. Our result is mostly simplified, but we can have a few more steps. Some mathematicians will inquire that the RHS be set to zero if the equation is truly simplified. Others will ask that any recurring term be factored out. All three equations beneath are in varying degrees of simplification. v(x2+y2)=y 5x2+5y2-y=0 5x2+y(5y-i)=0 This is a polar equation. Our goal is to arrive at an equation that only contains x and y terms. Looking at the equation above, let's first rearrange it so that the trigonometric terms are on the RHS. 3r-cos2(θ)=sin2(θ) 3r=sin2(θ)+cos2(θ) See anything familiar? The RHS can be simplified to 1 using a pythagorean identity. 3r=ane How tin can we turn the LHS into r2? We have two options: we tin either multiply both sides past r or square both sides. Multiplying both side by r would mean that the RHS cannot be easily converted. If we square both sides instead, the equation is easier to solve. (3r)ii=12 9r2=1 Now that we have an equation with terms that can exist converted easily, we can begin to substitute. 9r2=1 ix(x2+y2)=1 Finally, we combine like terms and simplify the equation. Notice that you lot will arrive at the equation for a circle with a radius of 1/iii. Wasn't 9r2=i or r=1/3 a simpler way to show it? This is why polar equations can be and so helpful! 9(x2+y2)=one x2+y2=1/9 This is a rectangular equation. Our goal is to go far at an equation that only contains r and θ terms. Converting from rectangular course to polar form is much easier! Looking at the equation above, we can grouping the second-order terms in training to convert them to r2. x2+3x+y2=6 (x2+y2)+3x=six Substitute for all x and y terms. (x2+y2)+3x=half-dozen r2+3rcos(θ)=half dozen Nosotros could take the simplification on stride further hither, simply that is not necessary. Both answers have been shown beneath. r2+3rcos(θ)=6 r(r+3cos(θ))=6 And there you have information technology! Follow our Five Step Process whenever converting Polar to Cartesian equations and soon enough it'll go second nature!Why do Polar Coordinates and Equations exist?
Footstep 1: Identify the form of your equation
Step 2: Land your goal
Stride 3: Examine your equation
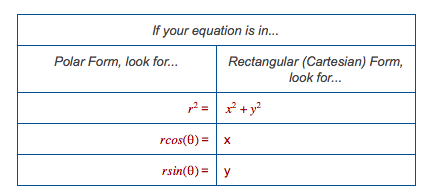
Step four: Substitute abroad!
Footstep 5: Combine like terms and complete squares (where needed)
Here are some examples!
Example 1: 5r=sin(θ)
Step one:
Step 2:
Step 3:
Step iv:
Step 5:
Example ii: 3r-cos2(θ)=sin2(θ)
Stride 1:
Stride 2:
Step 3:
Step 4:
Pace v:
Case three: x2+3x+y2=6
Stride ane:
Footstep ii:
Step 3:
Step 4:
Step 5:
How To Find Cartesian Equation,
Source: https://blog.cambridgecoaching.com/converting-polar-to-cartesian-equations-in-five-easy-steps
Posted by: hertzogdair1985.blogspot.com


0 Response to "How To Find Cartesian Equation"
Post a Comment